数据结构系列（1）之 二叉搜索树

本文将主要以动图方式展示二叉搜索树的结构，以及动态操作；但是对于基本的概念和性质则不会有过多的提及，如果想系统了解建议查看邓俊辉老师的《数据结构》课程；

一、结构概述

二叉树：融合了向量的静态操作（二分查找）和列表的动态操作（插入和删除）的优点；使得树成了应用广泛的数据结构；

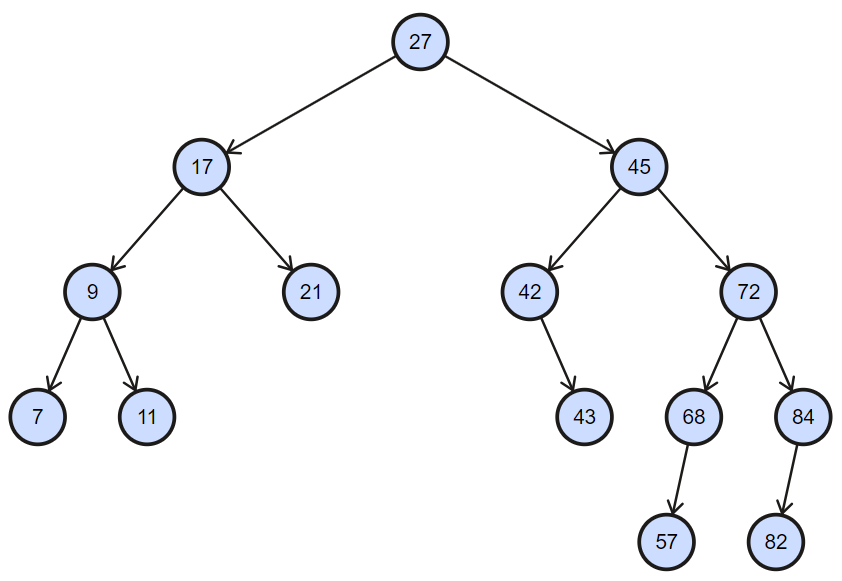
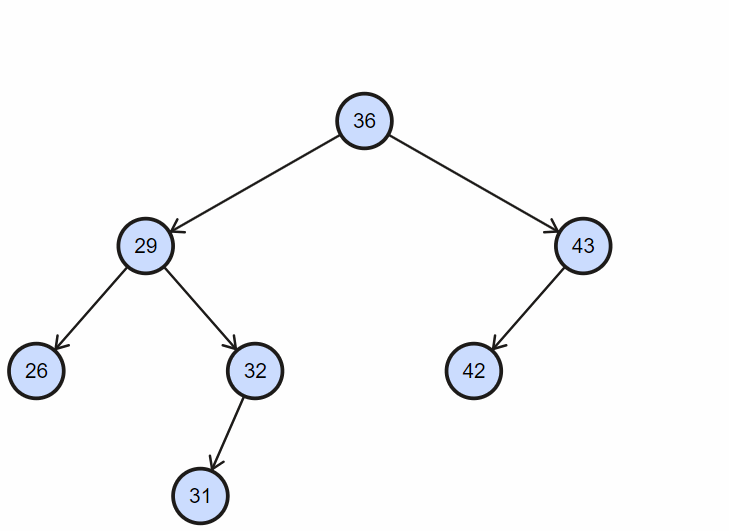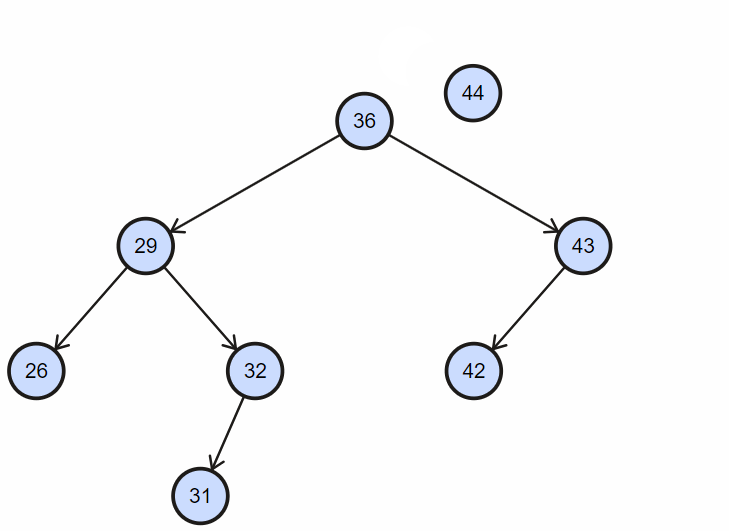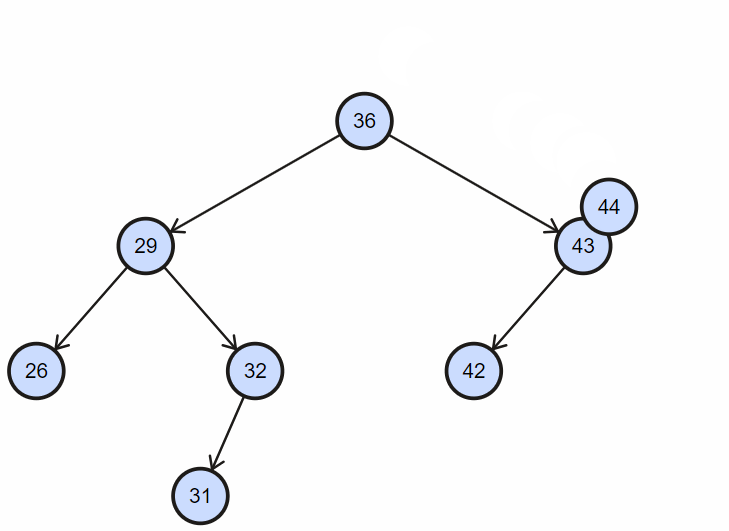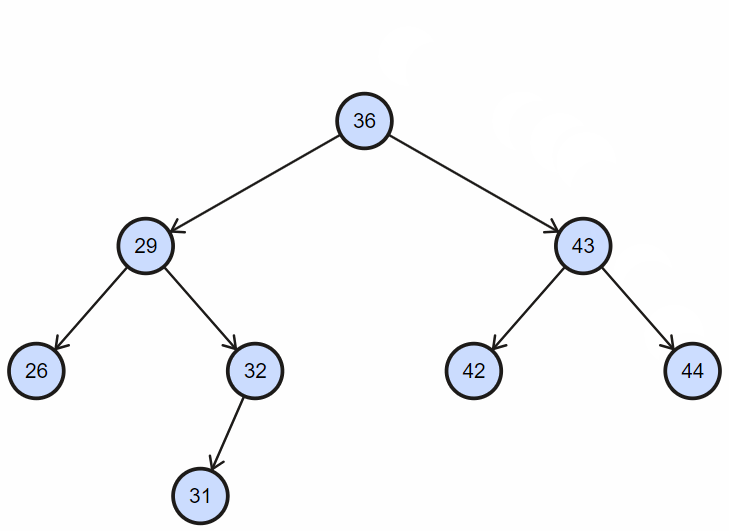
二叉搜索树：即顺序排列，可以搜索的树就是二叉搜索树；如下图所示；

忽略二叉树的大小，高度等信息的简版结构如下：

public class BST<T extends Comparable<? super T>> {  private BSTNode<T> root;  public BST() {root = null;}
  ...  
  class BSTNode<T extends Comparable<? super T>> {
    T key;
    BSTNode<T> parent;
    BSTNode<T> left;
    BSTNode<T> right;

    BSTNode(T key, BSTNode<T> parent, BSTNode<T> left, BSTNode<T> right) {      this.key = key;      this.parent = parent;      this.left = left;      this.right = right;
    }
  }
}

二、查找

根据二叉搜索树左孩子节点小于父节点，右孩子节点大于等于父节点，逐步深入查找；

实现如下：

private BSTNode<T> search(BSTNode<T> x, T key) {  if (x == null) return x;  int cmp = key.compareTo(x.key);  if (cmp < 0)    return search(x.left, key);  else if (cmp > 0)    return search(x.right, key);  else
    return x;
}public BSTNode<T> search(T key) {  return search(root, key);
}

更好的实现还应该记录查找失败的上一个节点，除此之外还可以实现查找最大值，最小值；

三、插入

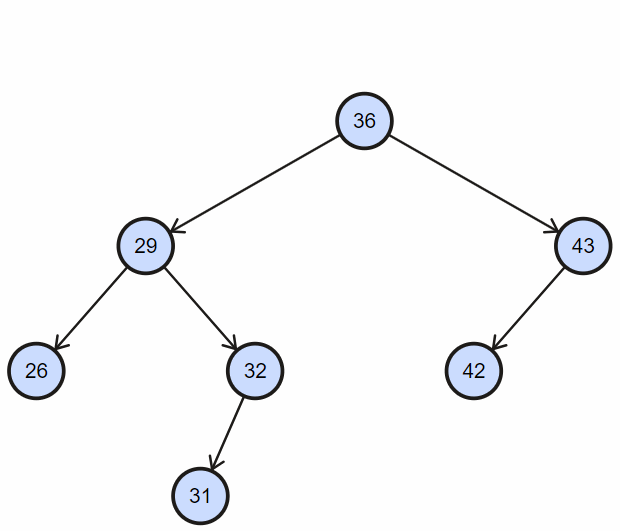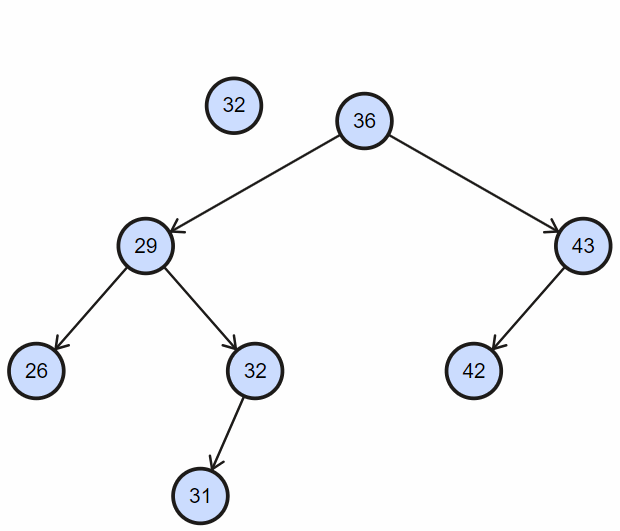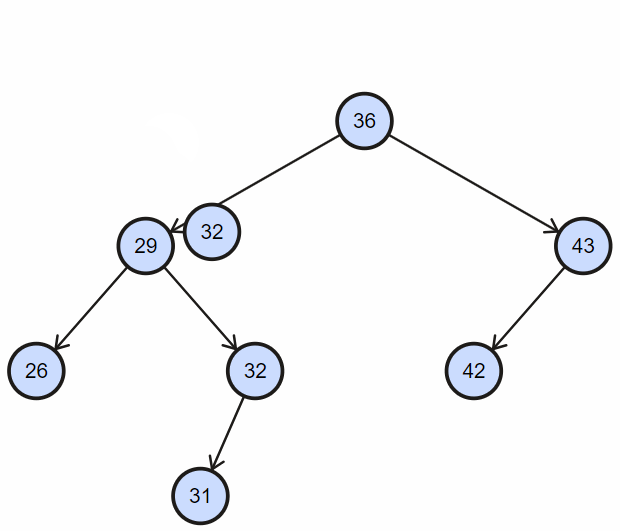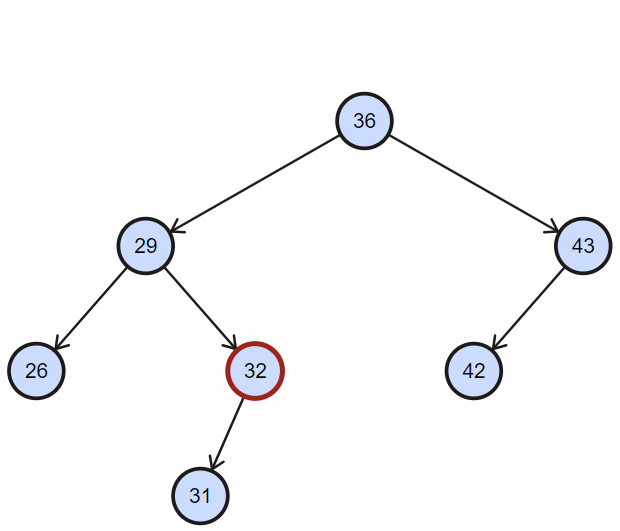
插入，同样根据 BST 的排序规则，先查找插入位置，然后确定插入节点的引用关系；

1. 插入不重复节点

2. 插入重复节点

3. 实现

如果有 size 等信息，则需要从节点向上依次更新祖先节点

private void insert(T key) {
  BSTNode<T> node = new BSTNode<T>(key, null, null, null);

  BSTNode<T> r = this.root;
  BSTNode<T> p = null;  int cmp;  // 查找插入位置
  while (r != null) {
    p = r;
    cmp = node.key.compareTo(r.key);    if (cmp < 0)
      r = r.left;    else
      r = r.right;
  }  if (p == null)    this.root = node;  else {
    node.parent = p;
    cmp = node.key.compareTo(p.key);    if (cmp < 0)
      p.left = node;    else
      p.right = node;
  }
}

四、删除

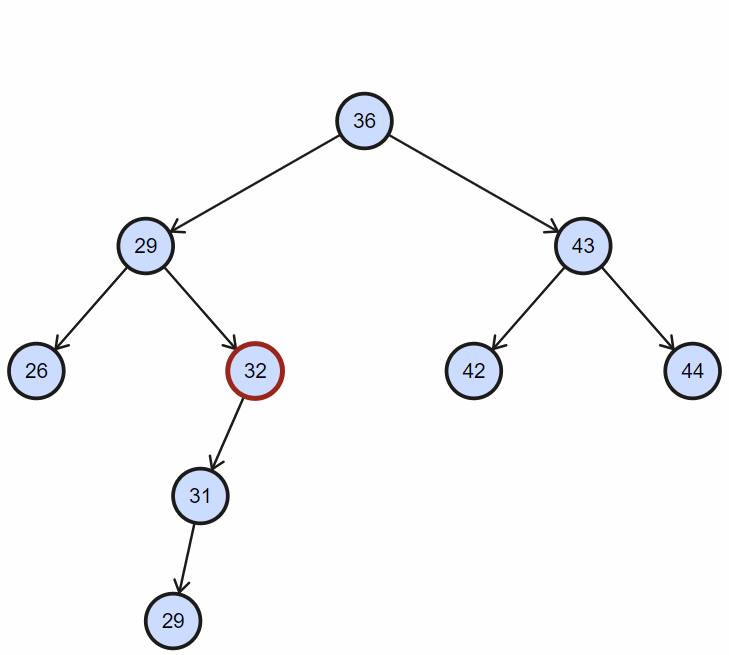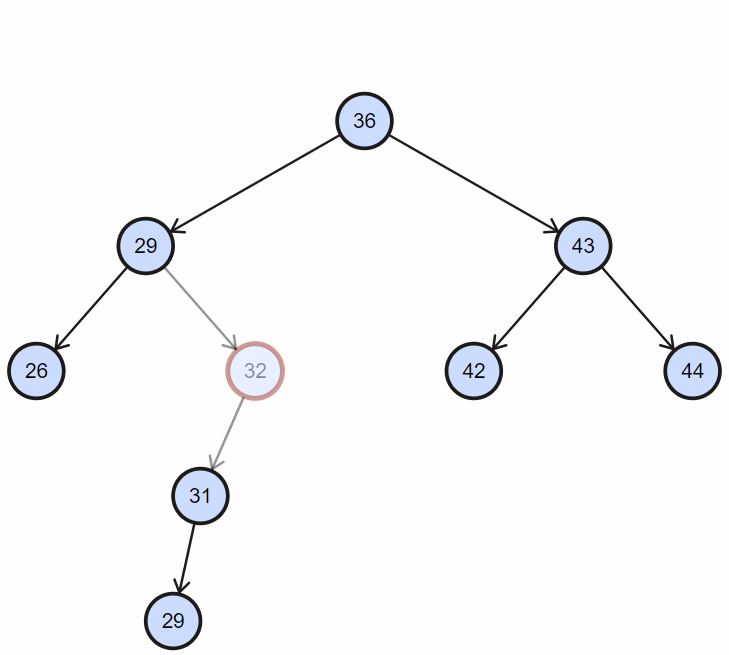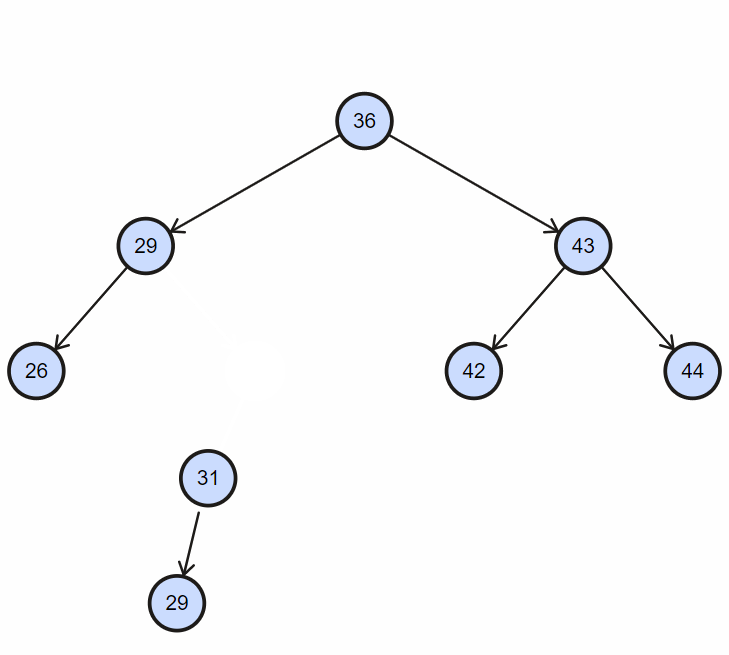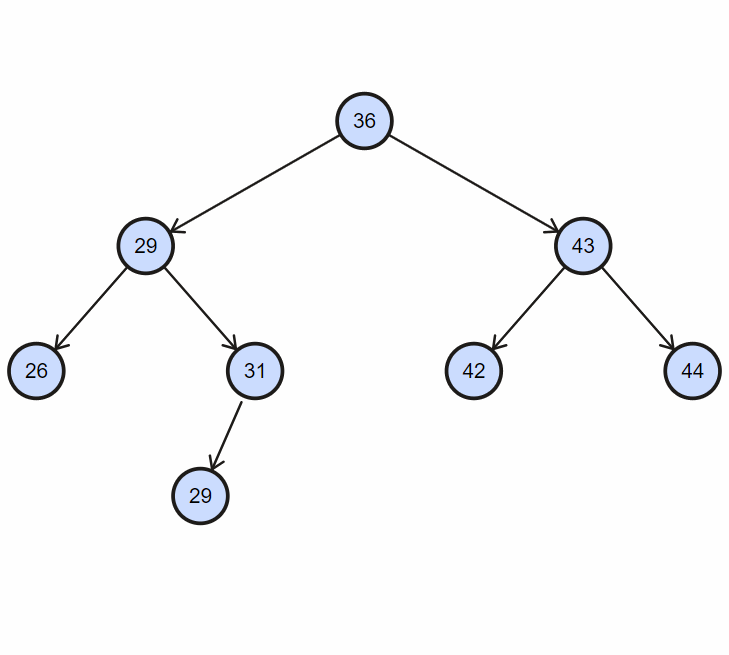
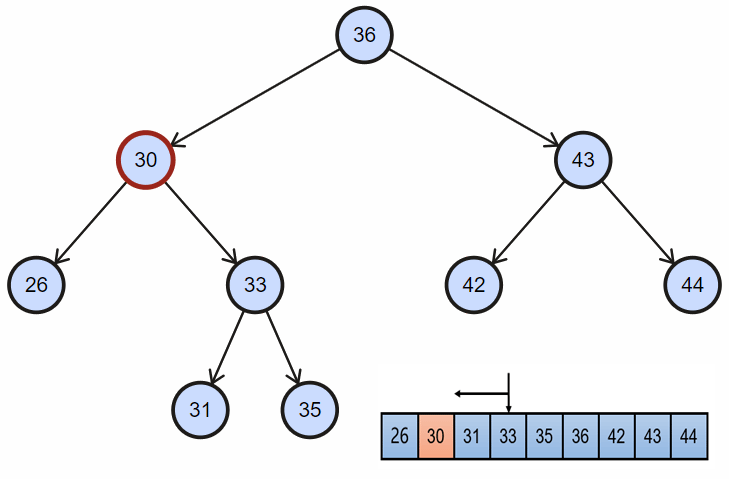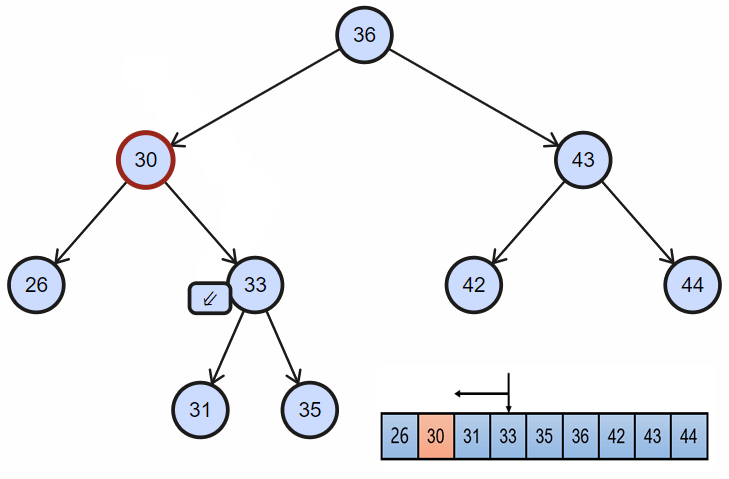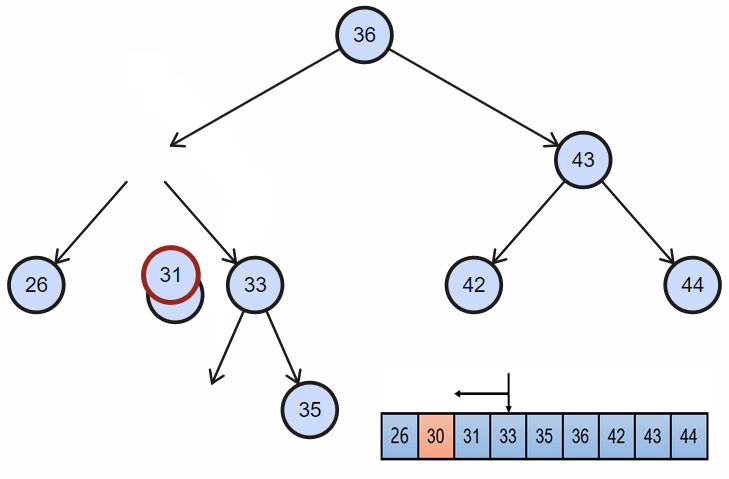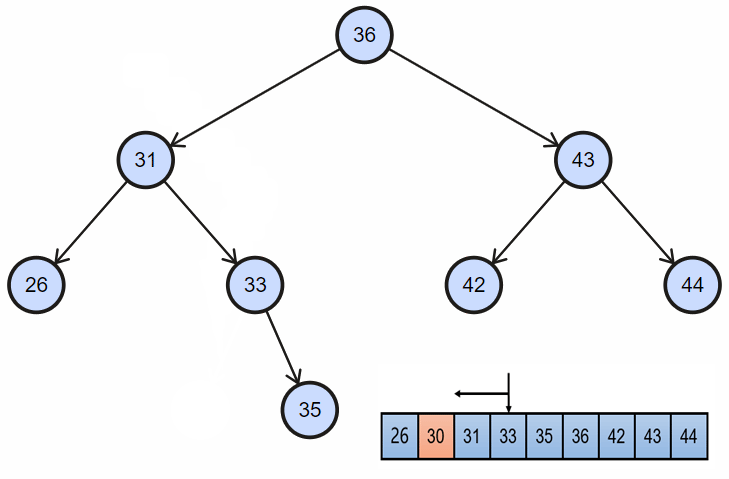
删除，同样首先需要查找该节点，然后删除；

1. 删除不完全节点

目标节点没有左孩子或者右孩子时，直接删除节点，然后令其后代代替；

2. 删除完全节点

当目标节点同时拥有左孩子和右孩子时：

• 需要首先找到目标节点的直接后继（自然顺序或者中序遍历顺序的下一个节点），目标节点右孩子一直往左；当然这里同样可以找目标节点的前驱节点替换；

• 然后交换目标节点和后继节点的位置；

• 最后同上面的删除一样，直接删除节点；

3. 实现

注意这里只是实现的一种，还可以是可前驱进行交换

public void delete(T key) {
  BSTNode<T> node = search(root, key);  if (node != null) delete(node);
  node = null;
}private BSTNode<T> delete(BSTNode<T> node) {  // 将完全节点和直接后继交换
  if (node.left != null && node.right != null) {
    BSTNode<T> succ = successor(node);
    node.key = succ.key;
    node = succ;
  }  // 将孩子节点的父亲接到祖父上
  BSTNode<T> child = node.left != null ? node.left : node.right;  if (child != null) child.parent = node.parent;  // 目标节点没有父节点则是根节点
  if (node.parent == null) root = child;    // 目标节点是左孩子时
  else if (node == node.parent.left) node.parent.left = child;    // 目标节点是右孩子时
  else node.parent.right = child;  return node;
}private BSTNode<T> successor(BSTNode<T> node) {
    BSTNode<T> succ = node.right;    while (succ.left != null) succ = succ.left;    return succ;
}

// 这里只是右子树中的直接后继；整个中序遍历中直接后继还可能是其父节点或者祖先节点；

五、前序遍历

对于一个数据结构而言，了解他最直接的方式就是遍历操作，而对于二叉树则有前、中、后、层次遍历四种；

1. 描述

• 首先遍历根节点

• 再一次递归遍历左子树和右子树；

具体过程如下：

2. 实现

// 递归实现private void travPre(BSTNode<T> tree) {  if (tree == null) return;

  System.out.print(tree.key + " ");
  travPre(tree.left);
  travPre(tree.right);
}// 迭代实现public void travPre2(BSTNode<T> tree) {
  Stack<BSTNode<T>> stack = new Stack<>();  while (true) {
    visitAlongVine(tree, stack);    if (stack.isEmpty()) break;
    tree = stack.pop();
  }
}private void visitAlongVine(BSTNode<T> tree, Stack<BSTNode<T>> stack) {  while (tree != null) {
    System.out.print(tree.key + " ");
    stack.push(tree.right);
    tree = tree.left;
  }
}

六、中序遍历

1. 描述

• 对于中序遍历，则首先遍历左子树

• 再遍历根节点

• 最后遍历右子树

具体过程如下：

2. 实现

// 递归实现private void travIn(BSTNode<T> tree) {  if (tree == null) return;

  travIn(tree.left);
  System.out.print(tree.key + " ");
  travIn(tree.right);
}// 迭代实现public void travIn2(BSTNode<T> tree) {
  Stack<BSTNode<T>> stack = new Stack<>();  while (true) {
    goAlongVine(tree, stack);    if (stack.isEmpty()) break;
    tree = stack.pop();
    System.out.print(tree.key + " ");
    tree = tree.right;
  }
}private void goAlongVine(BSTNode<T> tree, Stack<BSTNode<T>> stack) {  while (tree != null) {
    stack.push(tree);
    tree = tree.left;
  }
}

七、后序遍历

1. 描述

• 对于后序遍历，则首先遍历左子树

• 再遍历根右子树

• 最后遍历根节点

具体过程如下：

2. 实现

// 递归实现private void travPost(BSTNode<T> tree) {  if (tree == null) return;

  travPost(tree.left);
  travPost(tree.right);
  System.out.print(tree.key + " ");
}// 迭代实现public void travPost2(BSTNode<T> tree) {
  Stack<BSTNode<T>> stack = new Stack<>();  if (tree != null) stack.push(tree);  while (!stack.isEmpty()) {    if (stack.peek() != tree.parent)
      gotoHLVFL(stack);
    tree = stack.pop();
    System.out.print(tree.key + " ");
  }
}private void gotoHLVFL(Stack<BSTNode<T>> stack) {
  BSTNode<T> tree;  while ((tree = stack.peek()) != null)    if (tree.left != null) {      if (tree.right != null) stack.push(tree.right);
      stack.push(tree.left);
    } else
      stack.push(tree.right);
  stack.pop();
}

八、层次遍历

1. 描述

对于层次遍历而言就相对简单，即由上到下逐层遍历；

2. 实现

public void travLevel() {
  Queue<BSTNode<T>> queue = new LinkedList<>();
  queue.offer(root);  while (!queue.isEmpty()) {
    BSTNode<T> x = queue.poll();
    System.out.print(x.key + " ");    if (x.left != null) queue.offer(x.left);    if (x.right != null) queue.offer(x.right);
  }
}

总结

• 正是因为二叉树同时具有向量和列表的特性，所以他的使用场景非常广泛，当然他还有很多的变种，这些我们后续会继续讲到；

• 对于文中的动图大家可以在这个网站自行实验；http://btv.melezinek.cz/binary-search-tree.html

【版权声明】

作者：三枣

出处：https://www.cnblogs.com/sanzao/p/10444931.html

 本作品采用 知识共享署名-非商业性使用-相同方式共享 4.0 国际许可协议 进行许可。欢迎转载，但未经作者同意必须保留此段声明，且在文章页面明显位置给出原文连接。